Paper: Elastogranular Mechanics
Elastogranular Mechanics: Buckling, Jamming, and Structure Formation
David J. Schunter, Jr., Martin Brandenbourger, Sophia Perriseau, and Douglas P. Holmes,
Physical Review Letters, 120, 078002, (2018).
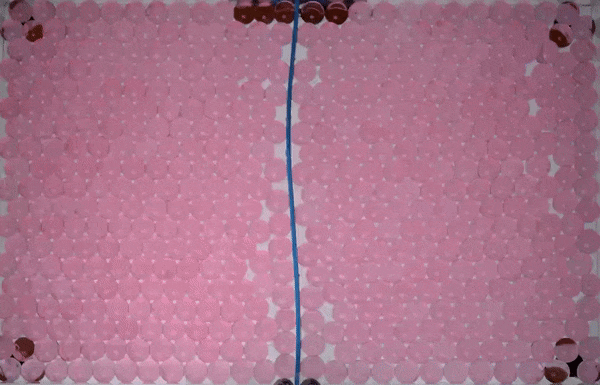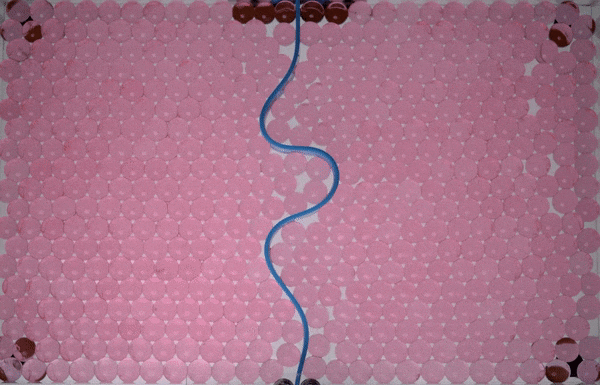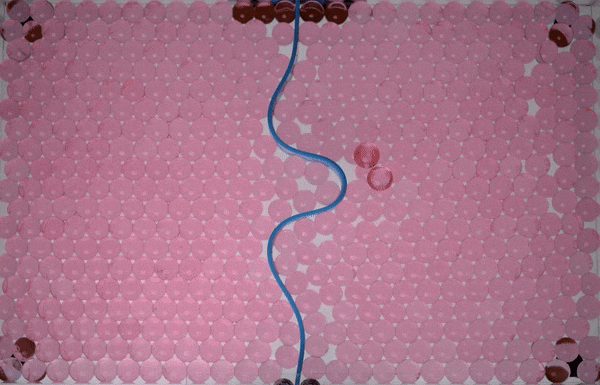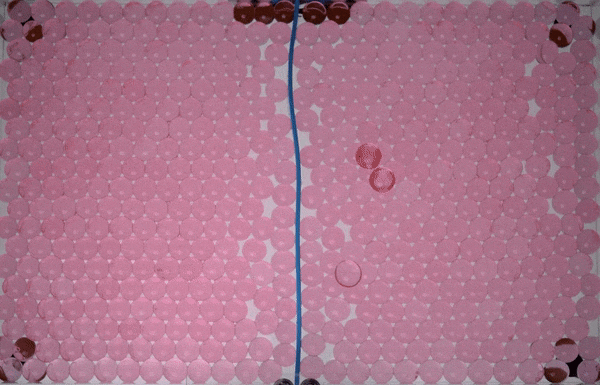
Confinement of a slender body into a granular array induces stress localization in the geometrically nonlinear structure, and jamming, reordering, and vertical dislodging of the surrounding granular medium. By varying the initial packing density of grains and the length of a confined elastica, we identify the critical length necessary to induce jamming, and demonstrate how folds couple with the granular medium to localize along grain boundaries. Above the jamming threshold, the characteristic length of elastica deformation is shown to diverge in a manner that is coupled with the motion and rearrangement of the grains, suggesting the ordering of the granular array governs the deformation of the slender structure. However, overconfinement of the elastica will vertically dislodge grains, a form of stress relaxation in the granular medium that illustrates the intricate coupling in elastogranular interactions.
Link: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.120.078002
Paper: Curvature-Induced Instabilities of Shells
Curvature-Induced Instabilities of Shells
Matteo Pezzulla, Norbert Stoop, Mark P. Steranka, Abdikhalaq J. Bade, and Douglas P. Holmes, Physical Review Letters, 120, 048002, (2018).
Induced by proteins within the cell membrane or by differential growth, heating, or swelling, spontaneous curvatures can drastically affect the morphology of thin bodies and induce mechanical instabilities. Yet, the interaction of spontaneous curvature and geometric frustration in curved shells remains poorly understood. Via a combination of precision experiments on elastomeric spherical shells, simulations, and theory, we show how a spontaneous curvature induces a rotational symmetry-breaking buckling as well as a snapping instability reminiscent of the Venus fly trap closure mechanism. The instabilities, and their dependence on geometry, are rationalized by reducing the spontaneous curvature to an effective mechanical load. This formulation reveals a combined pressurelike term in the bulk and a torquelike term in the boundary, allowing scaling predictions for the instabilities that are in excellent agreement with experiments and simulations. Moreover, the effective pressure analogy suggests a curvature-induced subcritical buckling in closed shells. We determine the critical buckling curvature via a linear stability analysis that accounts for the combination of residual membrane and bending stresses. The prominent role of geometry in our findings suggests the applicability of the results over a wide range of scales.
Link: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.120.048002
Paper: Extended Lubrication Theory
Extended lubrication theory: improved estimates of flow in channels with variable geometry
Behrouz Tavakol, Guillaume Froehlicher, Douglas P. Holmes, Howard A. Stone, Proceedings of the Royal Society A, 0234, (2017).
Abstract: Lubrication theory is broadly applicable to the flow characterization of thin fluid films and the motion of particles near surfaces. We offer an extension to lubrication theory by starting with Stokes equations and considering higher-order terms in a systematic perturbation expansion to describe the fluid flow in a channel with features of a modest aspect ratio. Experimental results qualitatively confirm the higher-order analytical solutions, while numerical results are in very good agreement with the higher-order analytical results. We show that the extended lubrication theory is a robust tool for an accurate estimate of pressure drop in channels with shape changes on the order of the channel height, accounting for both smooth and sharp changes in geometry.
Link: http://rspa.royalsocietypublishing.org/content/473/2206/20170234
Paper: Kirigami Actuators
Kirigami Actuators
Marcelo A. Dias, Michael P. McCarron, Daniel Rayneau-Kirkhope, Paul Z. Hanakata, David K. Campbell, Harold S. Park and Douglas P. Holmes, Soft Matter, 13, 9087-9802, (2017).
Abstract: Thin elastic sheets bend easily and, if they are patterned with cuts, can deform in sophisticated ways. Here we show that carefully tuning the location and arrangement of cuts within thin sheets enables the design of mechanical actuators that scale down to atomically-thin 2D materials. We first show that by understanding the mechanics of a single non-propagating crack in a sheet, we can generate four fundamental forms of linear actuation: roll, pitch, yaw, and lift. Our analytical model shows that these deformations are only weakly dependent on thickness, which we confirm with experiments on centimeter-scale objects and molecular dynamics simulations of graphene and MoS2 nanoscale sheets. We show how the interactions between non-propagating cracks can enable either lift or rotation, and we use a combination of experiments, theory, continuum computational analysis, and molecular dynamics simulations to provide mechanistic insights into the geometric and topological design of kirigami actuators.
Link: http://pubs.rsc.org/en/content/articlelanding/2017/sm/c7sm01693j#!divAbstract
BU Video: Slender Structures
What can we learn from the physics of a slinky? How these materials are inspiring engineers to embrace instability for advanced function. pic.twitter.com/mTcFHwIxnS
— Boston University (@BU_Tweets) September 6, 2017
Paper: Generalized Scaling Law of Adhesion
Revisiting the generalized scaling law for adhesion: role of compliance and extension to progressive failure
Ahmad R. Mojdehi. Douglas P. Holmes. David A. Dillard, Soft Matter, 13, 7529-7536, (2017).
Abstract: A generalized scaling law, based on the classical fracture mechanics approach, is developed to predict the bond strength of adhesive systems. The proposed scaling relationship depends on the rate of change of debond area with compliance, rather than the ratio of area to compliance. This distinction can have a profound impact on the expected bond strength of systems, particularly when the failure mechanism changes or the compliance of the load train increases. Based on the classical fracture mechanics approach for rate-independent materials, the load train compliance should not affect the force capacity of the adhesive system, whereas when the area to compliance ratio is used as the scaling parameter, it directly influences the bond strength, making it necessary to distinguish compliance contributions. To verify the scaling relationship, single lap shear tests were performed for a given pressure sensitive adhesive (PSA) tape specimens with different bond areas, number of backing layers, and load train compliance. The shear lag model was used to derive closed-form relationships for the system compliance and its derivative with respect to the debond area. Digital image correlation (DIC) is implemented to verify the non-uniform shear stress distribution obtained from the shear lag model in a lap shear geometry. The results obtained from this approach could lead to a better understanding of the relationship between bond strength and the geometry and mechanical properties of adhesive systems.
Link: http://pubs.rsc.org/en/content/articlelanding/2017/sm/c7sm01098b#!divAbstract
Talk: Museum of Science
Break it 'til You Make It: Engineering Shapes and Patterns
Gordon Current Science & Technology Center Stage, Blue Wing, Level 1
Saturday, August 26; 12:30 pm
Abstract: Not long ago, a structure losing stability led to failure and disaster. Instability makes rigid materials useless, so it was something that engineers had to design around. Soft materials, like rubbers, plastics, and gels, however, can bounce back from instability. The resilience of soft materials has enabled scientists to rethink the role of stability in the design of new materials. Douglas Holmes, an assistant professor of mechanical engineering at Boston University, explores the instabilities that cause skin to wrinkle, roots to tangle, toy poppers to jump, and we will discover how engineers design wearable electronics, make smart needles, and create ultra lightweight mechanisms.
Link: https://www.mos.org/live-presentations/guest-research-presentation
(Timestamped: https://goo.gl/58pLuv)
Paper: Friction of extensible strips
Friction of extensible strips: An extended shear lag model with experimental evaluation
Ahmad R. Mojdehi. Douglas P. Holmes. David A. Dillard, International Journal of Solids and Structures, 124, 125-134, (2017).
Abstract: The role of effective axial compliance on the frictional response of extensible strips is investigated, both experimentally and theoretically. A translational actuator pulled a steel sled resting on top of an elastic strip, bonded only at the leading edge of the sled, across a glass substrate. The friction force and local deformation along the length of the strips were measured using a force sensor and a camera, respectively. By increasing the effective axial compliance of the strip, the static friction force was found to decrease dramatically, while the kinetic friction force increased significantly. For sufficiently soft strips, there was no observable static peak, although there was a slope change in the force-displacement curve at the point where progressive slippage initiated at the leading edge. Possible mechanisms for permanent increase in the kinetic friction are discussed that could be implemented in systems where the kinetic friction is of significant importance. A theoretical model, somewhat analogous to an extension of the classical shear lag model to incorporate elastic-plastic interlayers, is proposed to predict the friction response as a function of effective compliance. The results obtained from the theoretical model are compared with experimental results and shown to be in good agreement. This study provides a better understanding of the effect of axial compliance on the frictional response of materials, paving the way for design and optimization of systems where the static and kinetic friction forces play an important role.
Link: http://www.sciencedirect.com/science/article/pii/S0020768317302858
Paper: Curvature-driven morphing of non-Euclidean shells
Curvature-driven morphing of non-Euclidean shells
Matteo Pezzulla, Norbert Stoop, Xin Jiang, Douglas P. Holmes, Proceedings of the Royal Society A, 473(2201), (2017).
Abstract: We investigate how thin structures change their shape in response to non-mechanical stimuli that can be interpreted as variations in the structure’s natural curvature. Starting from the theory of non-Euclidean plates and shells, we derive an effective model that reduces a three-dimensional stimulus to the natural fundamental forms of the mid-surface of the structure, incorporating expansion, or growth, in the thickness. Then, we apply the model to a variety of thin bodies, from flat plates to spherical shells, obtaining excellent agreement between theory and numerics. We show how cylinders and cones can either bend more or unroll, and eventually snap and rotate. We also study the nearly isometric deformations of a spherical shell and describe how this shape change is ruled by the geometry of a spindle. As the derived results stem from a purely geometrical model, they are general and scalable.
Link: http://rspa.royalsocietypublishing.org/content/473/2201/20170087
Among most downloaded articles in Extreme Mechanics Letters
Our paper "Buckling of elastic beams embedded in granular media" was among the top 5 most downloaded paper's in Elsevier's Extreme Mechanics Letters in 2016: